Analysis

Consider a rectangular duct of half widths $a$ and $b$ terminated in an infinite baffle, as shown in figure 3.4.

Figure 3.4: Geometry of infinitely flanged rectangular duct

Expressing (3.13) in rectangular coordinates for a rectangular duct of half-widths $a$ and $b$ gives:

$$Z_{nm} = \frac{i\omega\rho}{2\pi S^2} \int\limits_{-a}^{... ..._{-b}^{b} dy_0 \psi_m(x_0,y_0) \psi_n(x,y) \frac{e^{-ikh}}{h}$$ (3.26)

where

$$h = [(x-x_0)^2 + (y-y_0)^2]^{\frac{1}{2}},$$ (3.27)

and

$$\psi_n = \phi_{n_x}\sigma_{n_y},$$ (3.28)

with

$$\phi_{n_x} = N_{n_x}\cos \left(\frac{n_x \pi x}{a} \right),$$ (3.29)

$$\sigma_{n_y} = N_{n_y}\cos \left(\frac{n_y \pi y}{b} \right),$$ (3.30)

$$N_{n_x} = \left\{ \begin{array} {r@{\quad:\quad}l} 1 & n_x = 0, \\ \sqrt{2} & n_x > 0. \\ \end{array} \right.$$ (3.31)

Changing variables as in Swenson et al. [49] and Levine [51] to $\xi = x - x_0$, $\eta = x + x_0$, $\zeta = y - y_0$, and $\mu = y + y_0$ the integral becomes

$${Z_{nm} =}$$

$$\frac{i\omega\rho}{2\pi S^2} \int\limits_0^{2a} d\xi \int\limits_... ...hi_{m_{x0}} \int\limits_{-2b+\zeta}^{2b-\zeta}d\mu \sigma_{n_y} \sigma_{m_{y0}}$$ (3.32)

where

$$h = \sqrt{\xi^2 + \zeta^2}.$$ (3.33)

The quadruple integral can now be reduced to a double integral by performing integration by $\eta$ and $\mu$ analytically. The first step is to expand the cosines in equations (3.29) and (3.30):

$${\phi_{n_x} \phi_{m_{x0}}= N_n N_m \times}$$

$$\scriptstyle \Big[ \cos(\frac{n_x\pi\xi}{2a})\cos(\frac{m_x\pi\xi... ...in(\frac{m_x\pi\xi}{2a}) \cos(\frac{n_x\pi\eta}{2a})\sin(\frac{m_x\pi\eta}{2a})$$

$$\scriptstyle - \sin(\frac{n_x\pi\xi}{2a})\cos(\frac{m_x\pi\xi}{2a... ...c{m_x\pi\xi}{2a}) \sin(\frac{n_x\pi\eta}{2a})\sin(\frac{m_x\pi\eta}{2a}) \Big].$$ (3.34)

The second and third terms go to zero since we are integrating over a symmetric interval in $\eta$. Performing integration gives:

$${ G(n_x,m_x,\xi,a) = \int\limits_{-2a+\xi} ^{2a-\xi} d\eta \phi_{n_x} \phi_{m_{x0}} }$$

$$= N_{n_x} N_{m_x} (2a - \xi) \Bigg[ {\mathrm sinc}\left((n_x + m_... ...t(1-\frac{\xi}{2a}\right)\right) \cos\left(\frac{(n_x-m_x) \pi \xi}{2a}\right)+$$

$$\mbox{\hspace{3cm}} {\mathrm sinc}\left((n_x - m_x)\pi\left(1-\frac{\xi}{2a}\right)\right) \cos\left(\frac{(n_x+m_x) \pi \xi}{2a}\right) \Bigg].$$ (3.35)

The integral for the impedance is then:

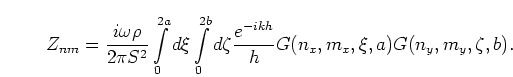$$Z_{nm} = \frac{i\omega\rho}{2\pi S^2} \int\limits_0^{2a} d\... ...eta \frac{e^{-ikh}}{h} G(n_x,m_x,\xi,a)G(n_y,m_y,\zeta,b).$$ (3.36)

Changing variables to $u = k\xi$ and $v = k\zeta$ means that the radiation impedance is expressed in terms of the dimensionless variables $ka$ and $kb$:

$$Z_{nm} = \frac{i \rho c}{2\pi S} \int\limits_0^{2ka} du ... ...1}{2}\right) G\left(n_y,m_y,\frac{v}{2kb},\frac{1}{2}\right).$$ (3.37)

Note that if we put $n_x = m_x = n_y = m_y = 0$ into equation (3.35) we obtain

$$G\left(n_x,m_x,\frac{u}{2ka},\frac{1}{2}\right) G\left(n_y... ... = 4\left(1-\frac{u}{2ka}\right)\left(1-\frac{v}{2kb}\right).$$ (3.38)

The radiation impedance from equation (3.37) is then identical to the radiation impedance of a rectangular piston in an infinite baffle [47,48,49,50,51] (note that most authors have used $a$ and $b$ as widths rather than half widths). Equation (3.37) has a singularity at the origin if $n_x = m_x$ and $n_y = m_y$ which must be removed if the radiation impedance is to be calculated by numerical integration. To do this the integral is first split into two parts:

$${Z_{nm} =}$$

$$\frac{i\rho c}{2\pi S} \int\limits_0^{2ka} du \int\limits_0^{2kb} dv \frac{(1 - \frac{u}{2ka}) (1 - \frac{v}{2kb})}{\sqrt{u^2+v^2}}$$

$$\mbox{\hspace{4cm}} \left[e^{-i\sqrt{u^2+v^2}} \frac{G(n_x,m_x,\f... ...frac{G(n_y,m_y,\frac{v}{2kb},\frac{1}{2})}{(1 - \frac{v}{2kb})} - f(n,m)\right]$$

$$+\frac{i\rho c}{2\pi S} \int\limits_0^{2ka} du \int\limits_0^{2kb} dv \frac{(1 - \frac{u}{2ka}) (1 - \frac{v}{2kb})}{\sqrt{u^2+v^2}} f(n,m)$$ (3.39)

where

$${f(n,m) = N_{n_x}N_{m_x}N_{n_y}N_{m_y} \times}$$

$$\left[{\mathrm sinc}((n_x+m_x)\pi) + {\mathrm sinc}((n_x-m_x)\pi)\right] \left[{\mathrm sinc}((n_y+m_y)\pi) + {\mathrm sinc}((n_y-m_y)\pi)\right].$$ (3.40)

The first part is non-singular and the singularity in the second half may be removed by integration, giving:

$${Z_{nm} =}$$

$$\frac{i\rho c}{2\pi S} \int\limits_0^{2ka} du \int\limits_0^{2kb} dv \frac{(1 - \frac{u}{2ka}) (1 - \frac{v}{2kb})}{\sqrt{u^2+v^2}} \times$$

$$\mbox{\hspace{4cm}} \left[e^{-i\sqrt{u^2+v^2}} \frac{G(n_x,m_x,\f... ...frac{G(n_y,m_y,\frac{v}{2kb},\frac{1}{2})}{(1 - \frac{v}{2kb})} - f(n,m)\right]$$

$$+ \frac{i\rho c}{2\pi S}\int\limits_0^{2ka} du \left(1 - \frac{u}{2ka}\right) \times$$

$$\mbox{\hspace{2cm}} \left[ \ln{\left(2kb + \sqrt{u^2+(2kb)^2}\right)} + \frac{u}{2kb} - \frac{1}{2kb}\sqrt{u^2 + (2kb)^2} \right] f(n,m)$$

$$+ \frac{i\rho c}{2\pi S} \left[-ka\ln{(2ka)} + \frac{3}{2}ka \right] f(n,m).$$ (3.41)

Equation (3.41) may be evaluated by numerical integration to provide the radiation impedance.